バイナリ
gui/img/shelf_filter.png
ファイルの表示
+ 24
- 0
gui/index.html
ファイルの表示
| @@ -2347,6 +2347,30 @@ double s_freq = .0625;</p> | |||
| <p class=desc>Configure one stage of the filter (0 to 3) with band reject (notch) | |||
| response. Q controls the width of rejected frequencies. | |||
| </p> | |||
| <p class=func><span class=keyword>setLowShelf</span>(stage, frequency, gain, slope);</p> | |||
| <p class=desc>Configure one stage of the filter (0 to 3) with low shelf response. | |||
| A low shelf filter attenuates or amplifies signals below the specified frequency. | |||
| Frequency controls the slope midpoint, gain is in dB and can be both | |||
| positive or negative. The slope parameter controls steepness of gain transition. | |||
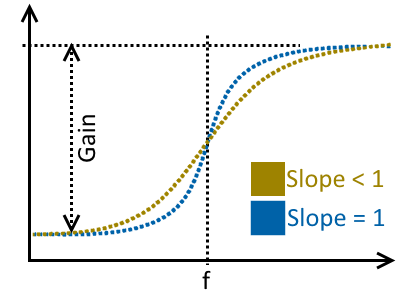
| A slope of 1 yields maximum steepness without overshoot, | |||
| lower values yield a less steep slope. See the picture below for a visualization | |||
| of the slope parameter's effect. | |||
| Be careful with positive gains and slopes higher than 1 as they introduce gain | |||
| (see warning below). | |||
| </p> | |||
| </p> | |||
| <p class=func><span class=keyword>setHighShelf</span>(stage, frequency, gain, slope);</p> | |||
| <p class=desc>Configure one stage of the filter (0 to 3) with high shelf response. | |||
| A high shelf filter attenuates or amplifies signals above the specified frequency. | |||
| Frequency controls the slope midpoint, gain is in dB and can be both | |||
| positive or negative. The slope parameter controls steepness of gain transition. | |||
| A slope of 1 yields maximum steepness without overshoot, | |||
| lower values yield a less steep slope. See the picture below for a visualization | |||
| of the slope parameter's effect. | |||
| Be careful with positive gains and slopes higher than 1 as they introduce gain | |||
| (see warning below). | |||
| </p> | |||
| <p align=center><img src="img/shelf_filter.png"></p> | |||
| <p class=func><span class=keyword>setCoefficients</span>(stage, array[5]);</p> | |||
| <p class=desc>Configure one stage of the filter (0 to 3) with an arbitrary | |||
| filter response. The array of coefficients is in order: B0, B1, B2, A1, A2. | |||
読み込み中…